Chapter 6 in Rau’s “The Beauty of Physics: Patterns, Principles, and Perspectives” discusses various forms of maps. Rau initially defines a map as the “representation of the positional layout of objects in a geographic landscape.” After the introduction of this basic definition, however, he expands this definition to include maps as “metaphors of language”. Many of the concepts addressed (mathematics, quantum physics, etc.) are not ones that are traditionally associated with maps – however, through the unifying theme of representation, Rau is able to explain their interconnection.

First, Rau discusses the role of maps in human history. Beginning with basic recognition of landmarks, early hominids developed the basic navigational techniques that evolved into modern mapping skills used by humans. Maps have played a key role in oceanic navigation for hundreds, if not thousands, of years. Early seafaring civilizations viewed maps as prized commodities, as they were able to give their viewers the knowledge of their relative position in the world. The novelty of maps has only increased since the invention of mapping technologies, such as GPS. Humans aren’t the only living things to use mapping – many living things use some form of mapping for navigation. Rau notes that long-distance migratory birds are expert navigators, and that “bees use a representational map on the walls of their hives…”

After discussing maps in human history, Rau goes on to explore maps in mathematics. The definition of a map is further modified for this context: The map is defined as “an association of one number or entity with another.” Based on this definition, mathematical functions are considered to be maps. Further, series of mathematical patterns that occurs at smaller and smaller scales, namely, fractals, are considered to be “iterated maps.” Similar to the advancement of mapping with technology, fractals can be easily generated using modern computer technology.

 

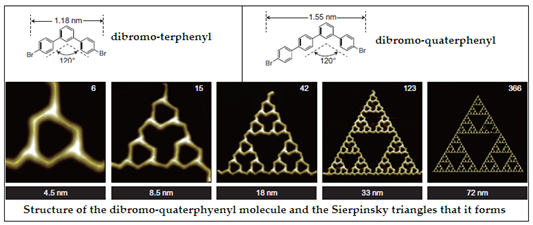
This view of fractals as maps is a very interesting perspective, especially considering my focus on fractal patterns for my final project. This viewpoint has provoked me to think about the meaning of my project from yet another perspective – the fractal pattern(s) and mirrors (symbolic of perspective) I incorporate into my creative display can potentially be viewed as a collective map of the observer’s perspective.

In the last section of this chapter, Rau delves into the application of maps to many complex concepts of physics. He claims that physics is able to map the relationship between two states of a single system. This concept, along with the examples Rau includes, are pretty confusing – he addresses extremely complex concepts such as spatial wave functions, the Bohm-Aharanov effect, and Feynman’s path-integral formulation. With the exception of basic quantum physics concepts (such as electron spin and the Schrödinger equation) that I studied last term in my General Chemistry course, the ideas presented in this part of the chapter are completely beyond my knowledge. One concept that sparked my interest is the existence of several dimension beyond the few that humans are familiar with. I found Rau’s brief mention of six-dimensional space very intriguing, yet impossible to comprehend – I’d love to have a discussion about multi-dimensional space, and learn how others attempt to comprehend this concept.